Correcting the correlation and covariance between two complex concepts
It may happen that in a study not only one complex concept is used but two or more are used. In this section we will discuss how in this case the correction of the correlation and covariance matrices can be performed. We start with the specification of the standardized model with two complex concepts sharing the same method.
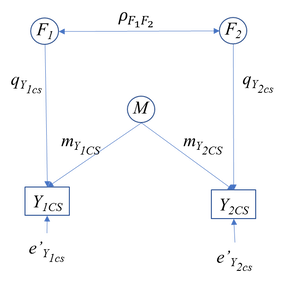
Given what has been derived for complex concepts and their composites scores, we can reduce the model to the one presented in Figure below.
Because for both complex concepts the quality coefficients are known and this figure is now not different from the situation discussed for two simple concepts, we can refer to the derivation there and conclude for the correlation between the two concepts that the correlation will be:
ρF1F2 = (ρY1cs Y2cs - mY1cs mY2cs) / qY1cs qY2cs
and the covariance is equal to
σF1F2 = σF1 ρF1F2 σF2
Because all these coefficients have been derived before in terms of the quality of the simple questions we can compute these correlations and covariances for any possible number of complex concepts.